Airy Rainbow Simulator
Airy Rainbow Simulator: A Comprehensive Analysis
Rainbows are mesmerizing natural phenomena that have fascinated humans for centuries. The interaction of light with water droplets in the atmosphere gives rise to these beautiful displays of color. While rainbows are a common sight, there are intricate details that often go unnoticed due to the limitations of our vision and the complexity of the underlying physics. In order to gain a deeper understanding of rainbows, scientists have developed advanced tools and simulations. One such tool is the Airy Rainbow Simulator, which allows us to explore the intricate details of rainbow formation and intensities.
Understanding Rainbow Intensities
When a plane light wave encounters a water droplet, it undergoes internal reflection and refraction. This interaction causes the outgoing wave to curve, leading to the formation of a rainbow. The intensity of the resulting rainbow and its supernumeraries, which are additional bands of color, can be calculated by determining the phase differences along the curved wavefront.
The Airy Rainbow Simulator is based on the work of George Biddell Airy, an English Astronomer Royal who made significant contributions to the field of optics in the 19th century. Airy approximated the shape of the scattered wavefront with a cubic form and developed an analytic expression for rainbow intensities using what are now known as Airy integrals or functions. His theory provides satisfactory predictions for white light rainbows and is computationally faster than more exact methods such as Mie theory.
The Functionality of AirySim
AirySim utilizes precomputed and stored Airy functions, which are derived through an ascending series expansion. These functions cover a wide range of arguments and are used to compute rainbows for specific parameters such as drop size, wavelength, and refractive index. For non-monodisperse droplets, AirySim employs a droplet population function that follows a normal distribution in radius. The simulator repeats calculations for different droplet radii and sums the intensities to obtain the final result.
To simulate white light rainbows, AirySim performs calculations for closely spaced wavelengths between 380 and 700 nm. The intensities at each angle are then weighted by a spectral solar radiance, which represents the distribution of solar energy across the visible spectrum. This allows for a realistic representation of the colors observed in natural rainbows.
Advantages and Limitations
The Airy Rainbow Simulator offers several advantages in the study of rainbow optics. Its use of Airy functions and efficient computation methods allows for faster calculations compared to more complex theories like Mie theory. This enables researchers to explore a wide range of scenarios and obtain valuable insights into the physics of rainbows.
However, it is important to note that AirySim has its limitations. The simulator assumes ideal conditions, such as perfectly spherical water droplets and a point source of light. In reality, variations in drop size and the finite angular size of the sun can blur the supernumeraries and affect the accuracy of the simulation. Additionally, while AirySim provides detailed calculations of rainbow intensities, it does not offer a user-friendly interface for general use.
Representation of Colors
Representing colors accurately is a challenging task in any simulation or visualization. AirySim addresses this issue by utilizing the CIE (Commission Internationale de l'Eclairage) and Bruton color models, which were specifically developed for the IRIS (Image Rendering for Immersive Scenes) system. These models aim to provide realistic color representations based on the properties of light and human perception.
Conclusion
The Airy Rainbow Simulator is a powerful tool that allows researchers and enthusiasts to delve into the intricate details of rainbow formation and intensities. By utilizing Airy functions and efficient computation methods, the simulator provides valuable insights into the physics behind rainbows. While it has certain limitations and lacks a user-friendly interface, AirySim offers a valuable resource for studying the complexities of atmospheric optics. With further advancements in simulation technology, we can continue to uncover the secrets of nature's most enchanting displays of light and color.
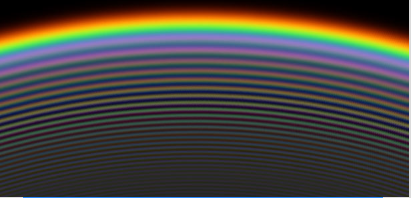
Bow from 0.75mm diameter drops illuminated by a distant point source. Supernumeraries like these are not seen in nature because they are blurred by the finite angular size of the sun and variations in drop size. AirySim simulation.
When a plane light wave interacts with a water drop the outgoing wave after a single internal reflection is curved. If the shape of the wave is somehow known then phase differences along it can be calculated and thus the intensity of the resulting rainbow and its supernumeraries. The English Astronomer Royal, George Biddell Airy (1801-1892), approximated the scattered wavefront shape with a cubic form and developed an analytic expression for the rainbow intensities in terms of what are now called Airy integrals or functions. Airy's theory gives satisfactory predictions of the observable features of white light rainbows(1) and is computationally very considerably faster than the exact predictions of Mie theory.AirySim precomputes and stores Airy functions for a whole range of arguments using an ascending series expansion(2). To compute a rainbow for a particular drop size, wavelength and refractive index(3), appropriate values of the Airy functions for each scattering angle are derived by interpolation of the stored values or, where necessary, additional direct computation. White light rainbows are obtained by repeating the calculation for closely spaced wavelengths between 380 and 700 nm and summing the intensities at each angle after weighting them by a spectral solar radiance(4).When simulations from non monodisperse droplets are required AirySim uses a droplet population function normally distributed in radius. All the above calculations have to be repeated for the different droplet radii and the intensities summed.Rainbows from the sun rather than plane parallel light are derived by convolving the angular intensities with a disk intensity function.Representation of colours is always problematic. AirySim uses the CIE and Bruton(5) colour models written for IRIS. Regrettably, AirySim is not available for download. The intentions were good bit I did not have the time to construct the necessary user friendly interface.
(1) Lee, R. L., "Mie theory, Airy theory, and the natural rainbow," Applied Optics 37, 1506-1519 (1998).http://www.usna.edu/Users/oceano/raylee/papers/RLee_papers.html (2) Abramowitz M. & Stegun I.A., Handbook of Mathematical Functions, Dover. (3) Water refractive indices are from IAPWS (International Association for the Properties of Water and Steam) which are in turn based on P. Schiebener, J. Straub, J. M. H. L. Sengers, J. S. Gallagher, "Refractive index of water and steam as function of wavelength, temperature and density," J. Phys. Ch. R.,19, 677-717, (1990).(4) Spectral solar radiances were those used by Raymond Lee(1) and kindly supplied by him in detailed tabular form. (5) Dan Bruton of Stephen F. Austin State University, "Color Science", http://www.physics.sfasu.edu/astro/color.html
Note: this article has been automatically converted from the old site and may not appear as intended. You can find the original article here.
Reference Atmospheric Optics
If you use any of the definitions, information, or data presented on Atmospheric Optics, please copy the link or reference below to properly credit us as the reference source. Thank you!
-
<a href="https://atoptics.co.uk/blog/airy-rainbow-simulator/">Airy Rainbow Simulator</a>
-
"Airy Rainbow Simulator". Atmospheric Optics. Accessed on July 27, 2024. https://atoptics.co.uk/blog/airy-rainbow-simulator/.
-
"Airy Rainbow Simulator". Atmospheric Optics, https://atoptics.co.uk/blog/airy-rainbow-simulator/. Accessed 27 July, 2024
-
Airy Rainbow Simulator. Atmospheric Optics. Retrieved from https://atoptics.co.uk/blog/airy-rainbow-simulator/.