OPOD - Multiple Image Mirage
OPOD - Multiple Image Mirage: A Fascinating Optical Phenomenon
Mirages have long fascinated observers with their mesmerizing optical illusions. Most commonly, hot road mirages display a single inverted image below the actual object. However, there are instances where these mirages exhibit multiple images, some upright and some inverted. These captivating phenomena were captured by Jari Luomanen in Finland, revealing the complexity and diversity of mirage formations.
Exploring the Unusual Mirage Display
In Luomanen's photographs, the road's slight undulations and temperature gradients contribute to the creation of a more elaborate mirage. While it is likely that many road mirages exhibit similar characteristics, we are often conditioned to expect only a single mirror reflection. The presence of multiple images challenges our preconceived notions and prompts us to delve deeper into understanding their origins.
Unraveling the Mirage Mystery
To comprehend the occurrence of multiple image mirages, we can turn to the topology theory of mirages. Rather than resorting to complex ray tracing calculations for specific cases, this theory provides a comprehensive framework for understanding the possibilities and limitations of mirage formations.
In this topological approach, we imagine that what we observe with our eyes is pasted onto a transparent sphere called the "image sphere." The eye or camera acts as the center of this sphere, while the object responsible for creating the mirage is envisioned on a more distant "object sphere." The refractions and curved rays occur between these two spheres.
The beauty of the topology approach lies in its simplicity. We need not concern ourselves with the intricacies of how or why ray paths curve. Instead, we focus on examining the relationship between the images pasted on the two spheres.
Visualizing Mirage Transformations
To better understand mirage transformations, we can create a rubbery, stretchable copy of the inner sphere, referred to as the "transfer surface." By inflating the transfer surface towards the outer object sphere, we aim to make the mirage images on the transfer sphere align perfectly with the single object sphere image. In this process, stretching and folding are allowed, but cutting, snipping, and discontinuities are forbidden.
By folding the transfer sphere, we can overlay and align the mirage images with the object. For example, three mirage images can be made to match the object image by folding the transfer sphere twice. If there were five images, an additional two folds would suffice. However, if there were four images, it would be impossible to achieve a congruent alignment.
This rubbery approach not only offers a playful exploration of mirage formations but also provides profound insights into their underlying rules and structure. For instance, it reveals that allowed mirages follow an erect, inverted, erect pattern. Mirages displaying an erect, erect, inverted sequence do not conform to smooth folding of the inner sphere.
Additionally, a significant revelation from this study is that complete mirages always consist of an odd number of images, including the "real" one. Attempting to fold the sphere to create an even number of images proves futile. Many mirages remain incomplete due to obstructions such as the ground or intervening air layers that obscure certain images from our view.
To gain a more comprehensive understanding of this intriguing topic, further exploration of related resources is highly recommended.
Mirages have fascinated scientists and observers alike for centuries. These captivating optical illusions continue to defy our expectations and challenge our understanding of light and atmospheric conditions. The occurrence of multiple image mirages offers a glimpse into the complexity of nature's optical phenomena and encourages us to explore further into the enigmatic world of atmospheric optics.

One mirage - Many images
Jari Luomanen (photos) captured these hot road mirages in Finland. All photos ©Jari Luomanen.
Unusually, they show multiple images and a careful look reveals that some are upright and some inverted.
Most hot road mirages, caused by light rays refracted in the density gradients between lower warm air and upper cool air layers, show only a single inverted image below the 'real' object.
Here, the slight road undulations and temperature gradients have conspired to give a more complete mirage. More road images are probably like this but we are conditioned to expect only a single mirror reflection.
How are they explained?
We could invoke special temperature gradients and do some complicated ray tracing calculations but they would only inform about one special case. Instead we can use the topology theory of mirages. That has already worked out the types of mirages that are possible and the ones that are impossible. See below......

The headlights reveal the multiple images.
They alternate as inverted, erect, inverted and so on...

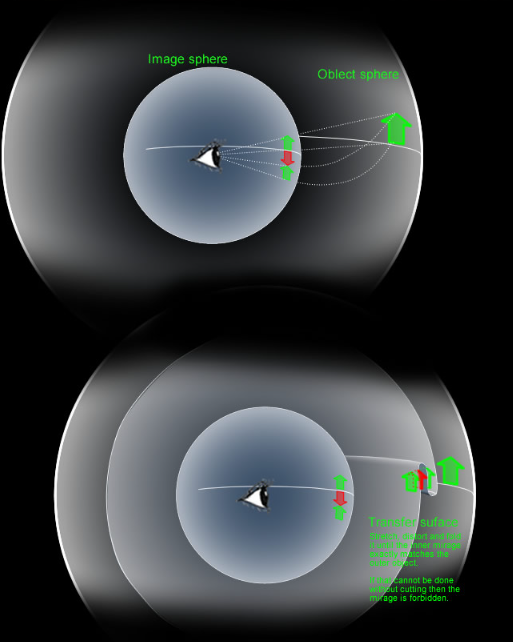
Topologically mirages can be represented by imagining that what we actually see by eye is pasted onto a transparent sphere. This is called the 'image sphere'. The eye or camera is at the sphere's centre.
The object creating the mirage can be imagined pasted onto a more distant 'object sphere'.
The refractions and curved rays occur between the two spheres. However, the beauty of this topology approach is that we do not have to worry about how or why the ray paths get curved. All we have to do is look at the relation between the images pasted on the two spheres.
The mirage from the inner sphere can be transformed into the object and vica-versa.
To do so make a rubbery stretchable copy of the inner sphere, Call it the transfer surface. Then inflate it so that it expands towards the outer object sphere. The aim is to make the mirage images on the transfer sphere exactly match the single object sphere image. In topology, stretching and folding is allowed - but in this case any cutting, snipping and discontinuities are forbidden.
Below left: The three mirage images are made to overlay each other and be congruent to the object by folding the transfer sphere twice. If there had been five images then another two folds would have done the trick. Had there been four images it would have been impossible.
The point of all this rubbery playing is that it generates deep insights into the underlying rules and structure of mirages. For example, allowed mirages are erect, inverted, erect,.. Mirages like erect, erect, inverted are not because they cannot be made by smooth folding of the inner sphere.
Another result is that in a complete mirage there are always an odd number of images (including the 'real' one). Try folding the sphere to make an even number! Many mirages are incomplete because the ground or an air layer cuts them off - we do not then see all the images.
For a more complete description see this page.


Note: this article has been automatically converted from the old site and may not appear as intended. You can find the original article here.
Reference Atmospheric Optics
If you use any of the definitions, information, or data presented on Atmospheric Optics, please copy the link or reference below to properly credit us as the reference source. Thank you!
-
<a href="https://atoptics.co.uk/blog/opod-multiple-image-mirage/">OPOD - Multiple Image Mirage</a>
-
"OPOD - Multiple Image Mirage". Atmospheric Optics. Accessed on December 22, 2024. https://atoptics.co.uk/blog/opod-multiple-image-mirage/.
-
"OPOD - Multiple Image Mirage". Atmospheric Optics, https://atoptics.co.uk/blog/opod-multiple-image-mirage/. Accessed 22 December, 2024
-
OPOD - Multiple Image Mirage. Atmospheric Optics. Retrieved from https://atoptics.co.uk/blog/opod-multiple-image-mirage/.